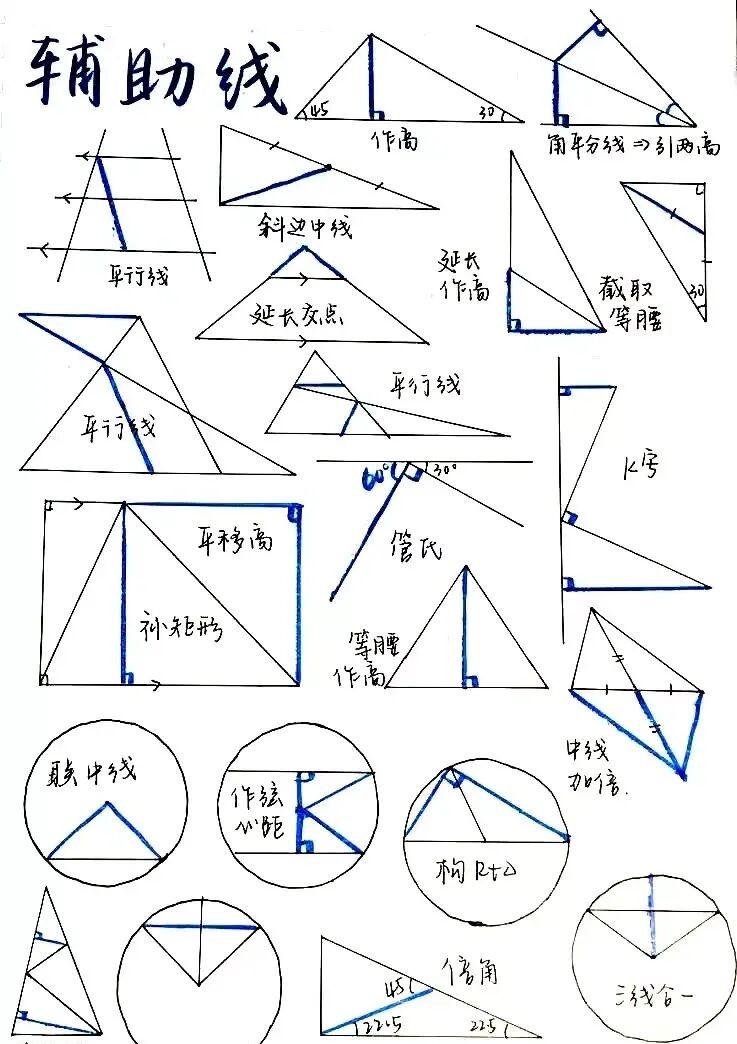
These 7 junior high school mathematics high score tips, before the middle school exam to master the "transparent", the first two and junior high school can be used, directly find the way of thinking:
First, the problem of adding or making a difference between the line segments is usually the highest frequency with the truncation complement method, and then the triangle is used to prove it.
Second, see the title for the midline:
1. The three-line combination of isosceles triangle.
2. Double the midline, that is, the multi-length midline method.
3. If the midline appears in the question of finding the third side of the value range, the triangle is also constructed by using the multi-length midline.
4. The median line theorem for triangles.
5). The midline on the hypotenuse of a right triangle is equal to half of the hypotenuse.
Third, see the 15 degrees 75 degree angle to pay attention to, 15 degrees can find a 30 degree angle at the outer corner, 75 degree angle can be found through the line segment relationship 45 degrees and 30 degree angles.
Fourth, find the length of the line segment.
1. Homogeneity and similarity ratio conversion.
2. Pythagorean theorem.
3. The projective theorem.
4. Equal area method.
5. The most valuable general drinking horse, Fermat point, Hu Bugui, etc.
Fifth, the folding problem will examine the relationship between the angle and the edge, such a question must find the full isoform and isosceles triangle in the figure to do the transformation, usually to find the target right triangle, set the requested edge as X, the second side is represented by an algebraic formula containing X, and the length of the third side is known.
Sixth, the topic of the angle of the isosceles triangle should be classified and discussed, and the known angle is the top angle or the bottom angle is classified and discussed.
Seventh, the equilateral triangle hand-in-hand model is almost used in all equilateral triangle problems, and the individual conclusions of the hand-in-hand model are considered when the method is not thought of.
For more junior high school mathematics level 2 conclusions and frequently tested mathematical models, manuscript notes, and error-prone questions, please click [Take a Look] below to get the full set.