Examination of the existence theorem of mathematical functions Limit Heine's theorem (principle of attribution)

Heine's theorem is a sufficient necessary condition for proving the existence of functional limits , and it develops from the relationship between the existence of the limits of a sequence of numbers and the limits of functions

Heine's theorem: let f be at u° (x. ;) is defined, the pre-existing condition is: for any inclusion

u°(x。 ;) and x. is the number series {} of the limit, the limits are all present and equal.

Let's analyze this theorem together.

Suppose that the graph of function f is shown in the figure

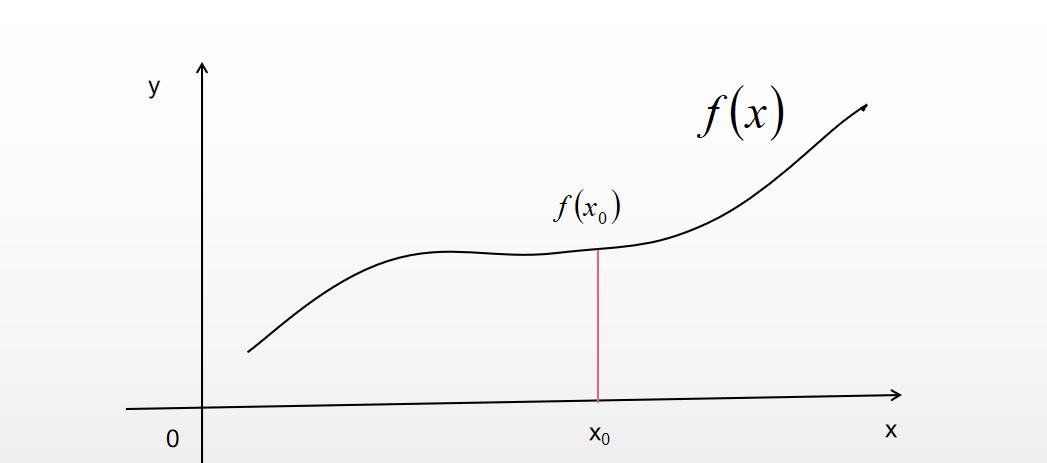
Function image

Around the points we can find infinitely more than one sequence of numbers,,...... And these sequences are considered to be the limit. Because on the axis of real numbers, each point on the axis of real numbers represents a real number, and each real number is densely distributed around an infinite number of real numbers, and these real numbers can form an infinite number of columns that are considered to be the limit.

When n ∞, there is

f()f( )

……

So, for anything contained in u°(x. ;) and x. is the number series {} of the limit, the limits are all present and equal.

This means existence.

Finally, we will prove this theorem based on the above analysis.

Proof: First we will prove the necessity of the theorem, which we now know exists

Set =A, then

According to the definition of the limit of the function, it is obtained that the pair >0, > 0, when 0 <<, there is

<e

Thus there will be an infinite number of sequences in ( the compactness theorem of the limit of the number series ) .

Finally, to prove the sufficiency of this theorem, we use the method of disproof.

Suppose ≠ A

According to the definition of the limit of the function, there is

> 0, to δ> 0, when 0 < <δ, there is

≥

Now let's take <δ

Order =, = 、、......、,

The limit of the sequence is, again, the arbitrariness according to it

Thus for any sequence of numbers that is considered to be the limit, there is ≠ A

This is related to the known condition "to anything contained in u°(x.). ;) and x. is the number series {} of the limit, the limits are all present and equal. "Contradictory.

So, there is