Indecisive quantum mechanics? Recently, scientists have used quantum mechanics to solve a famous mathematical problem dating back 243 years - the Euler 36 officer problem.
A mathematical problem that "has no solution" 243 years ago
The Euler 36 Officer Problem is a famous question posed by the Swiss mathematician Leonhard Euler in 1779. Euler envisioned that there were 6 corps with 6 officers each, and that the officers in each corps would have different ranks, and asked if the 36 officers could be arranged in a 6×6 phalanx with no duplicate corps and rank in each row and column? This problem can also be described as whether two 6th-order Latin squares can be orthogonal.
In 1900, the French mathematician Gaston Tarry listed all possible 6×6 square matrix arrangements, proving that the above 6×6 square matrix problems were unsolvable. In 1960, mathematicians used computers to prove that the problem was always solved when the number of corps and ranks was greater than 2 and not equal to 6. This means that phalanx problems such as 4×4, 5×5, 7×7 can be solved, and the 6×6 phalanx problem has become an "unsolvable mystery" that lasted for 243 years.
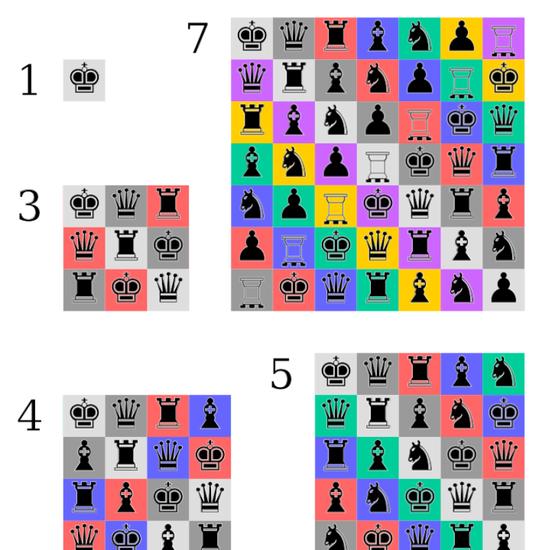
Solution to the 1st, 3rd, 4th, 5th, 7th rank phalanx officer problem, colors representing different corps, symbols representing different ranks, picture from Wikipedia
Quantum mechanics solves the Euler 36 officer problem
Since in the classical physical world, the Euler 36 officer problem has no solution. So in the world of quantum physics, can it be solved?
Suhail Rather of the Madras Institute of Technology (IITM) in India, Adam Burchardt of the Jagiellonian University (UJ) in Poland, and their colleagues proposed the above idea, which was studied under the conditions of quantum mechanics. They successfully demonstrated that as long as the officers could have a quantum hybrid state of rank and legion, the 36 officers could be arranged in a way that met Euler's standards. The results were recently published in the Physical Review Letters.
Image courtesy of Physical Review Letters
In the quantum version of the Euler 36 officer problem, the officer is formed by the superposition of ranks and corps, for example an officer can be a colonel of the Red Army or a lieutenant of the Blue Army. This version requires a definition to adjust, i.e. the two superimposed quantum states must be different.
The superposition between two quantum objects usually means that they are entangled, so their properties are interdependent and correlated. For example, if one officer is found to be a colonel, the other officer entangled with it should be a lieutenant.
From this, the researchers started with an approximate solution of the classical arrangement of 6×6, improved it into a quantum version by increasing the superposition of officers, and used a large number of calculations by computers to finally find a quantum solution to the Euler 36 officer problem.
Discovery of the "Absolute Maximum Entanglement (AME) State of Gold"
The researchers realized that the quantum solution to the problem was closely related to the quantum information processing problem involving the absolute maximum entanglement (AME) state. In the AME state, any pair of entangled qubits has a strong correlation. AME states are associated with quantum error correction, which refers to the recognition and correction of errors in quantum computing without actually reading out qubits.
Qubits have two possible readout states — 0 and 1 — but in principle, quantum objects can also have three or more states. Theorists have derived mathematical expressions for AME states for quantum objects of different sizes, but the AME states of four objects with six states, the AME (4, 6) state, remain elusive.
The quantum solution to Euler's 36 officer problem corresponds to the AME (4,6) state, picture from the paper
The researchers found a quantum solution to Euler's 36 officer problem, showing how to entangle four six-sided quantum dice, the so-called solution of the AME (4,6) state.
During the solution, the researchers found that the coefficients of the superposition of different quantum states were about equal to 1.618, which is the famous golden ratio, so they called the AME (4,6) state "golden AME state".
Barbara Kraus, a quantum information theorist at the University of Innsbruck in Austria, says finding the AME (4,6) state solves "a problem that some researchers have been working on for the past few years." Hoi-Kwong Lo, a quantum technology expert at the University of Toronto, sees the work as potentially meaningful, "And in my opinion, this argument seems credible." If the result is correct, I think it is very important and has implications for quantum error correction. ”