《程式設計之美: 求二叉樹中節點的最大距離》的另一個解法

http://www.cnblogs.com/miloyip/archive/2010/02/25/1673114.html

問題定義

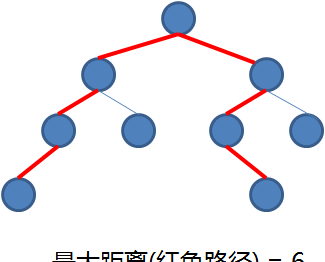
如果我們把二叉樹看成一個圖，父子節點之間的連線看成是雙向的，我們姑且定義"距離"為兩節點之間邊的個數。寫一個程式求一棵二叉樹中相距最遠的兩個節點之間的距離。

書上的解法

書中對這個問題的分析是很清楚的，我嘗試用自己的方式簡短覆述。

計算一個二叉樹的最大距離有兩個情況:

• 情況A: 路徑經過左子樹的最深節點，通過根節點，再到右子樹的最深節點。
• 情況B: 路徑不穿過根節點，而是左子樹或右子樹的最大距離路徑，取其大者。

隻需要計算這兩個情況的路徑距離，并取其大者，就是該二叉樹的最大距離。

我也想不到更好的分析方法。

但接着，原文的實作就不如上面的清楚 (源碼可從這裡下載下傳):

01

// 資料結構定義

02	struct NODE

03

{

04	NODE* pLeft;         // 左子樹

05	NODE* pRight;        // 右子樹

06	int nMaxLeft;        // 左子樹中的最長距離

07	int nMaxRight;       // 右子樹中的最長距離

08	char chValue;        // 該節點的值

09

};

10

11	int nMaxLen = 0;

12

13	// 尋找樹中最長的兩段距離

14	void FindMaxLen(NODE* pRoot)

15

{

16	// 周遊到葉子節點，傳回

17	if (pRoot == NULL)

18

{

19

return ;

20

}

21

22	// 如果左子樹為空，那麼該節點的左邊最長距離為0

23	if (pRoot -> pLeft == NULL)

24

{

25	pRoot -> nMaxLeft = 0;

26

}

27

28	// 如果右子樹為空，那麼該節點的右邊最長距離為0

29	if (pRoot -> pRight == NULL)

30

{

31	pRoot -> nMaxRight = 0;

32

}

33

34	// 如果左子樹不為空，遞歸尋找左子樹最長距離

35	if (pRoot -> pLeft != NULL)

36

{

37	FindMaxLen(pRoot -> pLeft);

38

}

39

40	// 如果右子樹不為空，遞歸尋找右子樹最長距離

41	if (pRoot -> pRight != NULL)

42

{

43	FindMaxLen(pRoot -> pRight);

44

}

45

46	// 計算左子樹最長節點距離

47	if (pRoot -> pLeft != NULL)

48

{

49	int nTempMax = 0;

50	if (pRoot -> pLeft -> nMaxLeft > pRoot -> pLeft -> nMaxRight)

51

{

52	nTempMax = pRoot -> pLeft -> nMaxLeft;

53

}

54

else

55

{

56	nTempMax = pRoot -> pLeft -> nMaxRight;

57

}

58	pRoot -> nMaxLeft = nTempMax + 1;

59

}

60

61	// 計算右子樹最長節點距離

62	if (pRoot -> pRight != NULL)

63

{

64	int nTempMax = 0;

65	if (pRoot -> pRight -> nMaxLeft > pRoot -> pRight -> nMaxRight)

66

{

67	nTempMax = pRoot -> pRight -> nMaxLeft;

68

}

69

else

70

{

71	nTempMax = pRoot -> pRight -> nMaxRight;

72

}

73	pRoot -> nMaxRight = nTempMax + 1;

74

}

75

76

// 更新最長距離

77	if (pRoot -> nMaxLeft + pRoot -> nMaxRight > nMaxLen)

78

{

79	nMaxLen = pRoot -> nMaxLeft + pRoot -> nMaxRight;

80

}

81

}

這段代碼有幾個缺點:

1. 算法加入了侵入式(intrusive)的資料nMaxLeft, nMaxRight
2. 使用了全局變量 nMaxLen。每次使用要額外初始化。而且就算是不同的獨立資料，也不能在多個線程使用這個函數
3. 邏輯比較複雜，也有許多 NULL 相關的條件測試。

我的嘗試

我認為這個問題的核心是，情況A 及 B 需要不同的資訊: A 需要子樹的最大深度，B 需要子樹的最大距離。隻要函數能在一個節點同時計算及傳回這兩個資訊，代碼就可以很簡單:

01	#include <iostream>

02

03	using namespace std;

04

05	struct NODE

06

{

07	NODE *pLeft;

08	NODE *pRight;

09

};

10

11	struct RESULT

12

{

13	int nMaxDistance;

14	int nMaxDepth;

15

};

16

17	RESULT GetMaximumDistance(NODE* root)

18

{

19	if (!root)

20

{

21	RESULT empty = { 0, -1 };    // trick: nMaxDepth is -1 and then caller will plus 1 to balance it as zero.

22	return empty;

23

}

24

25	RESULT lhs = GetMaximumDistance(root->pLeft);

26	RESULT rhs = GetMaximumDistance(root->pRight);

27

28	RESULT result;

29	result.nMaxDepth = max(lhs.nMaxDepth + 1, rhs.nMaxDepth + 1);

30	result.nMaxDistance = max(max(lhs.nMaxDistance, rhs.nMaxDistance), lhs.nMaxDepth + rhs.nMaxDepth + 2);

31	return result;

32

}

計算 result 的代碼很清楚；nMaxDepth 就是左子樹和右子樹的深度加1；nMaxDistance 則取 A 和 B 情況的最大值。

為了減少 NULL 的條件測試，進入函數時，如果節點為 NULL，會傳回一個 empty 變量。比較奇怪的是 empty.nMaxDepth = -1，目的是讓調用方 +1 後，把目前的不存在的 (NULL) 子樹當成最大深度為 0。

除了提高了可讀性，這個解法的另一個優點是減少了 O(節點數目) 大小的侵入式資料，而改為使用 O(樹的最大深度) 大小的棧空間。這個設計使函數完全沒有副作用(side effect)。

測試代碼

以下也提供測試代碼給讀者參考 (頁數是根據第7次印刷，節點是由上至下、左至右編号):

view source print ?

01	void Link(NODE* nodes,  int parent,  int left,  int right)

02

{

03	if (left != -1)

04	nodes[parent].pLeft = &nodes[left];

05

06	if (right != -1)

07	nodes[parent].pRight = &nodes[right];

08

}

09

10	void main()

11

{

12	// P. 241 Graph 3-12

13	NODE test1[9] = { 0 };

14	Link(test1, 0, 1, 2);

15	Link(test1, 1, 3, 4);

16	Link(test1, 2, 5, 6);

17	Link(test1, 3, 7, -1);

18	Link(test1, 5, -1, 8);

19	cout <<  "test1: " << GetMaximumDistance(&test1[0]).nMaxDistance << endl;

20

21	// P. 242 Graph 3-13 left

22	NODE test2[4] = { 0 };

23	Link(test2, 0, 1, 2);

24	Link(test2, 1, 3, -1);

25	cout <<  "test2: " << GetMaximumDistance(&test2[0]).nMaxDistance << endl;

26

27	// P. 242 Graph 3-13 right

28	NODE test3[9] = { 0 };

29	Link(test3, 0, -1, 1);

30	Link(test3, 1, 2, 3);

31	Link(test3, 2, 4, -1);

32	Link(test3, 3, 5, 6);

33	Link(test3, 4, 7, -1);

34	Link(test3, 5, -1, 8);

35	cout <<  "test3: " << GetMaximumDistance(&test3[0]).nMaxDistance << endl;

36

37	// P. 242 Graph 3-14

38	// Same as Graph 3-2, not test

39

40	// P. 243 Graph 3-15

41	NODE test4[9] = { 0 };

42	Link(test4, 0, 1, 2);

43	Link(test4, 1, 3, 4);

44	Link(test4, 3, 5, 6);

45	Link(test4, 5, 7, -1);

46	Link(test4, 6, -1, 8);

47	cout <<  "test4: " << GetMaximumDistance(&test4[0]).nMaxDistance << endl;

48

}

你想到更好的解法嗎?