For the magic formula, ancient and modern Chinese and foreign countries have done too much research, it can be said that there are too many books to finish. But when it comes to the magic square, the only thing we think of may be the third-order magic square like the following picture, and that's all. In fact, even the simplest third-order magic formula, there are many interesting things. Here's everything for you.
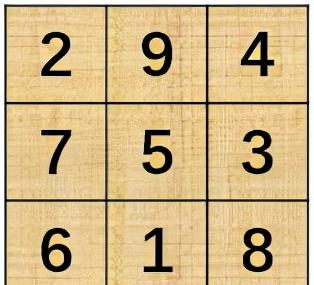
The nature of the third-order magic square
If each row from left to right is regarded as a 3-digit number, from right to left it is also regarded as a 3-digit number, then there is
294+753+618=492+357+816=1665
Not only that, but also the sum of their squares is equal, i.e
Of course, the above pairs also hold true
276+951+438=672+159+834=1665
Numbers on the corners = half of the sum of two numbers diagonally adjacent, as shown in the following figure
The construction of the magic square
For the third-order magic square, we do not need complex calculations, we can easily write. As early as ancient China, there were nine palaces to fill in the law: the righteousness of the nine palaces, the law to the spirit turtle, two and four as the shoulder, six eight as the foot, left seven right three, wear nine shoes one, five in the center.
The Southern Song Dynasty mathematician Yang Hui also summarized the method: nine sons diagonally arranged, up and down to easy, left and right phase change, four-dimensional prominence.
Now to the focus of this article, the construction method of the third-order magic square summarized by the mathematician Yang Hui mentioned above can actually be applied to the construction of all odd-order magic squares. The key is to translate up and down, left and right. Take the 5th order magic square as an example, step by step to explain.
First of all, it also needs 1 to 25 diagonal rows
A 5th-order magic square will then be constructed inside the dashed box below, while the numbers outside the dotted box will only need to be simply translated up and down, left and right.
Pan up and down:
Pan left and right:
At this point, the dotted box is the 5th order magic square.
The above method applies to the construction of all odd magic squares. Of course, there are many ways to construct the magic square, but the above method does not require complex calculations, nor does it require rote memorization, only need to be arranged obliquely, then panned, and easily written. Archimedes once said, give me a fulcrum and I can pry up the earth! At this point, you can also proudly say that no matter how large the number, I can easily write the magic square of this order, as long as there is a large enough paper. (Even-numbered magic squares will not be repeated here, interested can consult the relevant information.) )
There are many interesting things about the magic square, and the exquisite magic square constructed by Chinese and foreign scholars will be updated later, so stay tuned.
Like this article welcome to follow, favorite, retweet. At the same time, I also hope to pay attention to the WeChat public account - the number of things, to share more interesting mathematical knowledge for you.