Children who have just entered high school have not yet escaped from the clutches of the collection, and will immediately "hang" under the big tree of functions. So why do functions scare so many people? In addition to the complexity and variety of its problem types, I personally think that it is mainly because there are many abstract concepts involved in functions, such as the concept of functions, the concept of monotonicity of functions.... Although junior high school students are also exposed to some mathematical concepts, in fact, those concepts are not strictly defined, but only through some specific affairs or specific situations to let everyone feel. When you enter high school, you are exposed to many abstract concepts at once, and if you can't understand these concepts, many topics are difficult to write. Thus, let the high school children complain about the knowledge of functions.
To understand the concept of functions, let's first understand the history of functions.
One: The origin of the function
The word "function" used in Chinese mathematical books is a transliteration. Li Shanlan, a mathematician of the Qing Dynasty, translated "function" into "function" when he translated the book "Algebra" (1859).
The ancient Chinese characters "letter" and "contain" are common, and both have the meaning of "containing". The definition given by Li Shanlan is: "The formula contains heaven and is a function of heaven." "In ancient China, four words, heaven, earth, man, and thing were used to represent four different unknowns or variables. The meaning of this definition is: "Whereever the formula contains the variable x, the equation is called the function of x." So "function" means that the formula contains variables. The exact definition of the equation we are talking about refers to an equation that contains unknowns. However, in China's early mathematical monograph "Nine Chapters of Arithmetic", the meaning of the equation refers to a jointly established primary equation containing multiple unknown quantities, that is, the so-called system of linear equations [1].
Two: early concepts
In the seventeenth century, Galileo, in Two New Sciences, contained almost all of the concept of functions or variable relations, expressing the relationships of functions in the language of words and proportions. Around 1637, Descartes had noticed the dependence of one variable on another in his analytic geometry, but since it was not yet realized to refine the concept of functions, no one had yet clarified the general meaning of functions until Newton and Leibniz established calculus in the late 17th century, and most functions were studied as curves.
In 1673, Leibniz first used "function" for "power", and later he used this word to represent the abscissa, ordinate, tangent length and other geometric quantities of points on the curve. At the same time, Newton used "flow" in his calculus discussion to represent the relationship between variables [1].
THREE: Eighteenth century
In 1718 John Bernoulli defined the concept of functions on the basis of the Leibniz concept of functions: "a quantity composed of any one variable and any form of constant." He meant that all the equations of the variable x and the constant were called x functions, and stressed that functions should be represented by formulas.
In 1748, Euler defined a function in his book Introduction to Infinite Analysis as " A function of a variable is an analytic expression consisting of some number or constant of that variable and any one of them." He called the definition of the function given by John Bernoulli an analytic function, further distinguished it into algebraic and transcendental functions, and also considered "arbitrary functions." It is not difficult to see that Euler's definition of the function is more general and broader than John Bernoulli's definition.
In 1755, Euler gave another definition: "If certain variables depend in a certain way on other variables, that is, when the latter variables change, the previous variables also change, we call the preceding variables a function of the latter variables." ” [1]
IV: Nineteenth century
In 1821, Cauchy gave a definition from the beginning of the definition of variables: "There is a certain relationship between certain variables, and when the value of one of the variables is given and the values of the other variables can be determined with it, the initial variable is called an independent variable, and the other variables are called functions." In Cauchy's definition, the word argument appears first, noting that there is not necessarily a parsing expression for a function. However, he still believes that functional relationships can be expressed in multiple parsing equations, which is a big limitation.
In 1822 Fourier discovered that certain functions could be represented by curves, by a formula, or by multiple equations, thus ending the debate over whether the concept of a function was represented by a single equation and pushing the understanding of functions to a new level.
In 1837 Dirichlet broke through this limitation and decided that how to establish the relationship between x and y was irrelevant, and he broadened the concept of functions, pointing out: "For every definite value of x on a certain interval, y has a definite value, then y is called a function of x." This definition avoids the description of dependencies in function definitions and is accepted by all mathematicians in a clear way. This is what is often referred to as the classical function definition.
After Canto's set theory occupied an important place in mathematics, Oswald Wiebrun gave modern function definitions with the concepts of "set" and "correspondence", further concretized the correspondence, domain and value domain of functions through the concept of sets, and broke the limit of "variables are numbers", variables can be numbers or other objects [1].
Five: Modern concepts
F. Hausdorff in 1914 Hausdorff defines functions in the Compendium of Set Theory with the ambiguous concept of "ordinal couple", which avoids the ambiguous concepts of "variables" and "correspondences". Kuratowski's use of the set concept to define "preamble" in 1921 made Hausdorff's definition rigorous.
A new modern function in 1930 was defined as " If for any element x of set M , there is always an element y determined by set N corresponding to it , then a function is defined on set M , denoted f." Element x is called the independent variable, and element y is called the dependent variable" [1].
Now that we understand the history of the concept of functions, let's talk about the concept of functions in detail.
The traditional definition, that is, the concept of functions that we are exposed to in junior high school: in general, in a process of change, it is assumed that there are two variables x, y, if for any x there is a uniquely determined y and it corresponds to it, then x is called the independent variable, y is the function of x. The range of values for x is called the domain of the function, and the range of values for the corresponding y is called the range of values of the function [1].
The modern definition, that is, the use of the language of the set of the set described: let A, B is a non-empty set of numbers, if according to some definite correspondence f, so that for any number x in set A, there is a unique definite number y in set B and its correspondence, then it is called the mapping f: A→ B is a function from set A to set B, denoted as y = f(x), xεA, where x is called an argument, y is called the function of x, set A is called the domain of the function, y corresponding to x is called the function value, and the set of function values { f(x)|xεA} is called the value range of the function, and f is called the corresponding law. Among them, the definition domain, value domain, and correspondence law are called the function three-element definition domain, and the value domain, the corresponding law is called the three elements of the function [1].
The learning of function concepts officially opens the prelude to our learning of functions. Later, you will learn the properties and images of functions, six basic elementary functions: power-finger pairs, three trigonometry, and their new functions obtained by adding, subtracting, multiplying, and dividing composite operations, and derivative functions. It can be said that functions occupy half of the mathematical knowledge in high school.
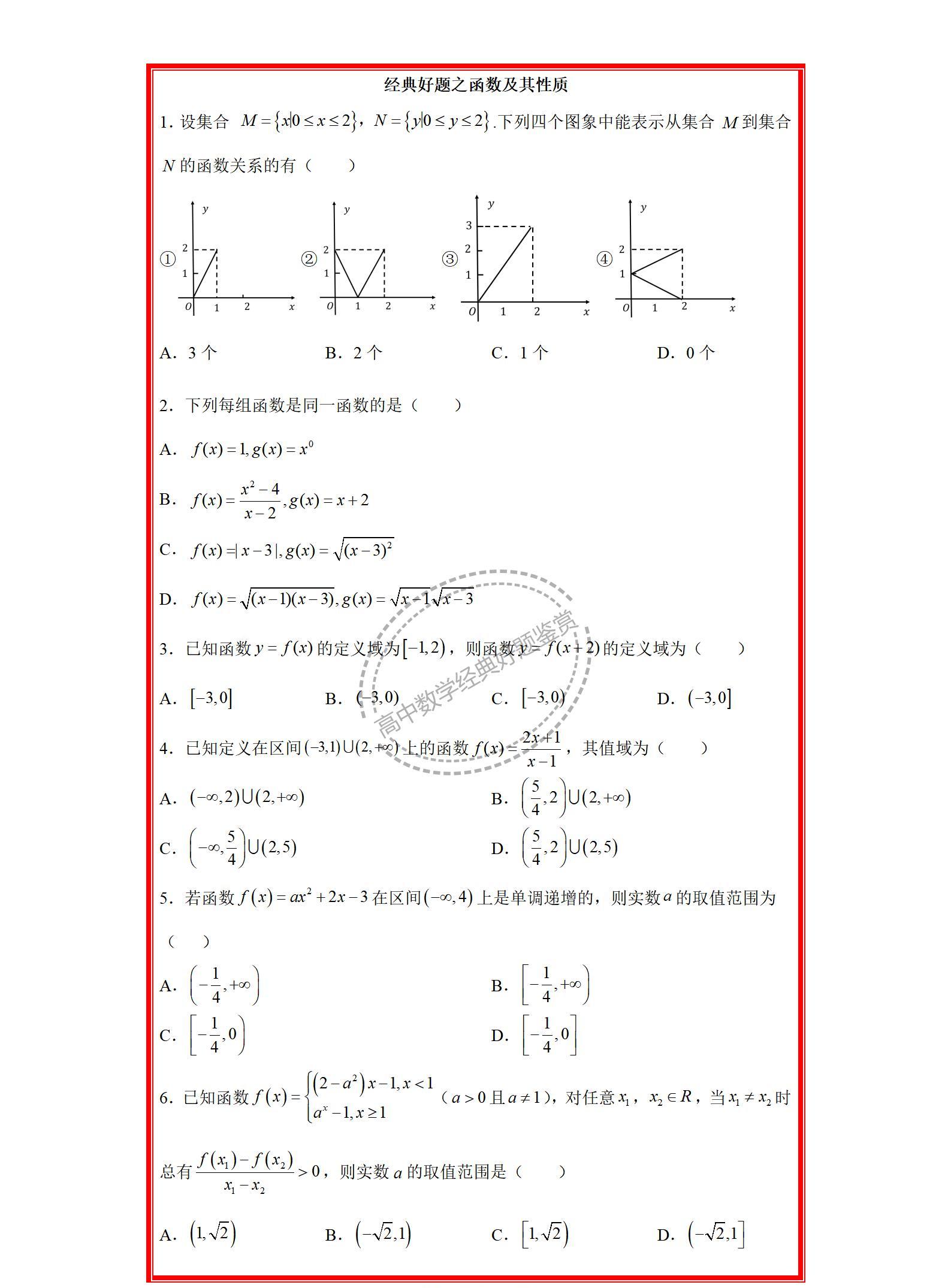
The following are some of the classic topics in the concept and properties of functions, and I believe that through the training of these topics, you can have a deeper understanding of the concept of functions and their properties.
Resources
1. Tang Rena, Bai Shuyan, etc. Advanced Mathematics (Engineering): Tsinghua University Press, Beijing Jiaotong University Press, 2004:5